

The nature of the roots of equation
ax2+bx+c=0 is determined by:
a) synthetic division
b) Sum of roots
c) Discriminant
d) Product of roots
Cube roots of -1 are:
a) -1 , -w , -w2
b) -1 , w , -w2
c) -1 , -w , w2
d) 1 , -w , -w2
If b2-4ac>0 and is a perfect square , then roots of ax2+bx+c=0 are:
a) irrational , equal
b) Rational , unequal
c) Rational , equal
d) irrational , unequal
Sum of the cube roots of unity is
a) 0
b) 1
c) -1
d) 3
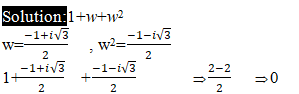
Product of the cube
roots of unity is
a) 0
b)1
c) -1
d) 4

If b2– 4ac<0 and is a
perfect square , then roots of ax2+bx+c=0 are:
a) irrational , equal
b) Imaginary
c) Rational , equal
d) irrational , unequal